how to find profit function

Profit Function

A profit function is a mathematical relationship between a firm's total profit and output. It equals total revenue minus total costs, and it is maximum when the firm's marginal revenue equals its marginal cost.

A firm's profit increases initially with increase in output. It is because the firm's average cost falls initially due to economies of scale. For example, at higher output level, the firm is able to avail bulk discounts, its workers specialize in different tasks, etc. Further, with increase in output the firm's fixed costs are spread out over more units causing the average fixed cost to fall. However, the firm's profit ultimately starts to fall when the law of diminishing returns kicks in. Since at least one output is fixed in the short-run, the return to the variable input falls as its units increase. It causes the average total cost to rise and the profit to fall. Due to this mechanism, the firm's profit curve is an inverted parabola as shown in the graph below.

Example

Let's consider a firm whose total revenue and total cost functions are given below:

$$ \text{TR}\ =\ \text{90Q}\ -\ \text{2Q}^\text{2} $$

$$ \text{TC}\ =\ \text{200}\ +\ \text{10Q}+\text{2Q}^\text{2} $$

Since profit (π) is defined as total revenue (TR) minus total costs (TC), the profit function can be written as follows:

$$ \pi=\text{TR}\ - \text{TC} $$

$$ \pi=\text{90Q}\ -\ \text{2Q}^\text{2}\ - (\text{200} + \text{10Q}+\text{2Q}^\text{2}) $$

A bit of simplification gives us this equation:

$$ \pi=\text{80Q}\ -\ \text{4Q}^\text{2}\ - \text{200} $$

Revenue, Cost and Profit Graphs

If we plug in different values of Q starting with 1, in the total revenue, total cost and profit function, we get the following table:

Q	TR	TC	Profit
1	88	212	(124)
2	172	228	(56)
3	252	248	4
4	328	272	56
5	400	300	100
6	468	332	136
7	532	368	164
8	592	408	184
9	648	452	196
10	700	500	200
11	748	552	196
12	792	608	184
13	832	668	164
14	868	732	136
15	900	800	100
16	928	872	56
17	952	948	4
18	972	1,028	(56)
19	988	1,112	(124)
20	1,000	1,200	(200)
21	1,008	1,292	(284)
22	1,012	1,388	(376)
23	1,012	1,488	(476)
24	1,008	1,592	(584)

If we plot the data above, we get the following curves:

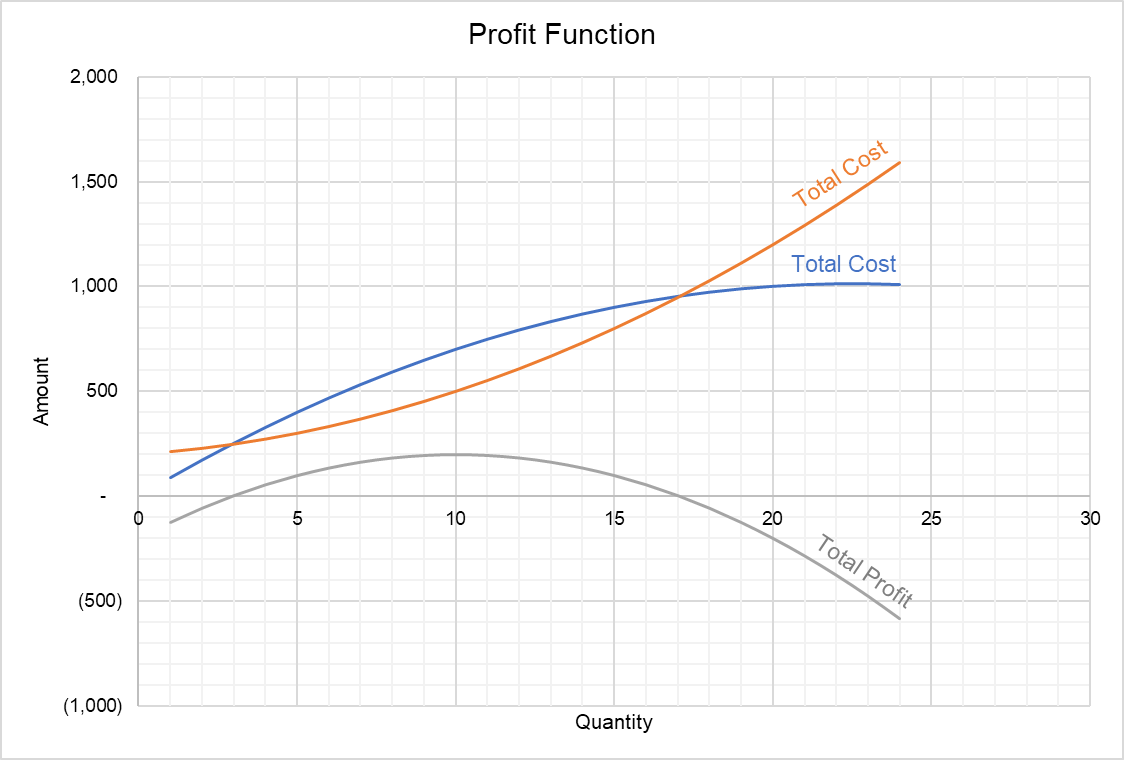
The profit curve plotted above is arrived at by subtracting the total cost curve from the total revenue curve. It starts at a point below the x-axis because the firm is making losses at low output (because even its fixed costs are not recovered). The curve initially slopes upwards, reaches a peak when quantity produced is 10 and then starts to fall. The profit is maximized at output of 10.

Since profit maximization occurs at a point at which the marginal revenue (MR) equals marginal cost (MC), we can verify that it is indeed maximized at Q = 10 by solving the marginal revenue and marginal cost functions (which are obtained by differentiating the total revenue and total cost functions with respect to Q).

$$ \text{MC}\ =\ \text{4Q}\ +\ \text{10}=\text{MR}\ =\ \text{90}\ -\ \text{4Q} $$

$$ \text{4Q}\ +\ \text{4Q}=\text{90}\ - \text{10} $$

$$ \text{Q}=\text{10} $$

by Obaidullah Jan, ACA, CFA and last modified on Feb 15, 2019